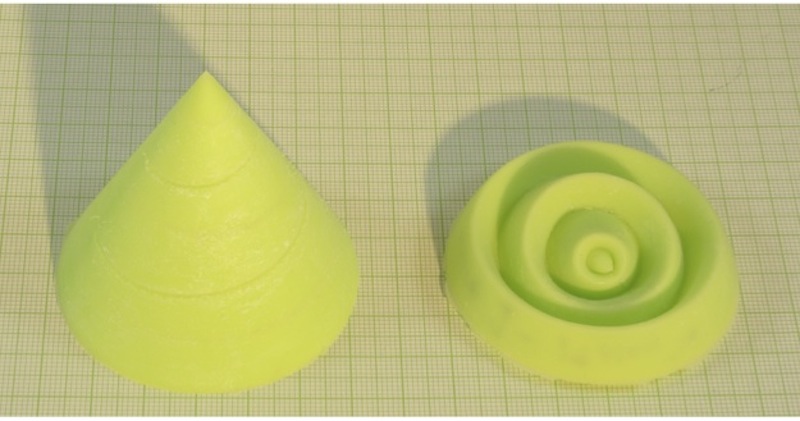
A new mathematical rule explains how simple, 3D curved surfaces — such as domes or saddles — can be folded and snapped into new positions or to form different structures.
Typically, snapping metal in half isn't a useful operation, but some objects could benefit from such innovative folding techniques. For instance, parts of a satellite need to collapse down for storage during launch but then quickly expand in space. Future robots could be more practical if they are able to reconfigure their arms without the need of moving parts. As such, understanding how to bend materials smoothly or snap them quickly could enable more efficient mechanical designs, said Arthur Evans, a postdoctoral researcher in the Department of Mathematics at the University of Wisconsin-Madison.
"There's a lot of math behind how you can fold flat things," Evans told Live Science. "There's a whole lot less [research] about how you can fold nonflat things." [Top 10 Inventions that Changed the World]
Origami artists usually fold flat sheets of paper to create shapes or structures. But folding materials with curves (such as dome- or saddle-shaped objects) usually means the finished product will be stiffer and stronger. This is similar to how folding a flat pizza slice into a cylinder-type shape helps keep the slice rigid.
The Venus flytrap is a domelike plant with leaves that are shaped like clamshells. When a fly brushes past the plant's sensitive hairs, it quickly folds the dome back together, snapping shut (like a spring mechanism without springs).
Engineers have used this snapping technique to build satellite airfoils that can collapse and expand, and to design tiny spherical particles that lock together. But researchers don't yet have theories to explain when or why it happens, Evans said.
Robert Lang, a physicist-turned-origami artist, published one of the earliest studies on folding nonflat surfaces in the journal The Mathematical Intelligencer in 2012. The research showed how to take paper curved in the 3D shape of a saddle (akin to a Pringles chip) and fold it into a crane.
In their new study, Evans and his colleagues found a general mathematical rule that explains whether a curved surface will either snap or bend smoothly when folded. The rule takes into account only the geometric shape of an object, not its material or size.
"It looks like we can get a lot of information just by looking at the geometry," Evans said. [Images: The World's Most Beautiful Equations]
To understand the mathematical rule, imagine a cylinder and a straight piece of wire. If the wire can wrap along the cylinder and doesn't deform it in any way, then you can fold the cylinder along that curve without snapping it.
If instead the wire bends tightly around the cylinder so that it strains to straighten out, then it will pull the cylinder and expand it slightly. If a curve pulls on any curved surface like this, the curve will snap when folded.
"The equations cover [folding] any kind of surface you could possibly think of," Evans said.
To experimentally test this rule, the team looked at three so-called shell shapes that mathematically represent all the different cases of curvature: the cylinder, the sphere and the spiral-staircase-shaped helicoid. The researchers found that, in general, the sphere always snaps while the helicoid bends along two special paths and snaps everywhere else.
Evans and his colleagues created 3D-printed models made out of dental rubber and plastic and strategically poked the models to examine how they deformed from different forces at different distances.
The researchers have not yet demonstrated any applications for the theory, but since the rule depends only on the shape of the surface, it can be applied to any material of any size, they said.
For example, at the microscopic scale, Evans speculated that knowing which curves snap quickly could someday help researchers create tiny snapping cells or capsules that could mix together liquids, such as drugs going into the human body, faster than mixing methods available today.
"They put together an elegant theory," Ashkan Vaziri, an engineering researcher at Northeastern University in Boston, who was not involved in the study but has studied such shapes, told Live Science.
Now, Evans and his colleagues said they are thinking about how to use their findings to design structures that can collapse down and lock into place, such as new, collapsible satellite airfoils. Engineers have been making locking structures that take advantage of bending or snapping for a while, but knowing a rule for such structures before they are designed would be more efficient, Evans said. Engineers could then pre-crease any curved object in just the right spots so that when it's pushed or slapped, it snaps or slowly bends into a different, predesigned configuration.
But for now, researchers only know for sure what happens to a single fold.
"It gets pretty complicated pretty fast," Evans said.
One of the next steps might be to investigate how to connect multiple folds together to create more-complicated structures, the researchers said. In the future, scientists might also investigate how to get structures to automatically bend or snap without being pushed or slapped.
The study is available on arXiv, a preprint server for science research, and was published online Thursday (Aug. 20) in the journal Proceedings of the National Academy of Sciences.
Follow Live Science @livescience, Facebook & Google+. Original article on Live Science.
