Zeno’s Paradox: Understanding Convergent & Divergent Series

In the fifth century B.C., the Greek philosopher Zeno of Elea attempted to demonstrate that motion is only an illusion by proposing the following paradox:

Achilles the warrior is in a footrace with a tortoise, but Achilles has given the tortoise a 100-meter head start. If Achilles runs 10 times as fast as the tortoise, by the time he catches up to the tortoise’s starting point, the tortoise will have advanced another 10 meters. It occurs to Achilles that the next time he catches up to where the tortoise is now, the tortoise will again have advanced … and this will be the case over and over to no end. By this logic, Achilles will never catch the tortoise!

Today we know that this paradox — Zeno created several that dealt with space and time — has nothing to do with motion being illusory, but we still talk about it because it introduced some interesting math that wouldn’t receive thorough treatment until the 17^th century A.D., when Gottfried Leibniz invented calculus. Even though the number of points where Achilles catches up to where the tortoise was last is infinite, the sum between all those points is finite. We call this phenomenon a “convergent series.”

A simpler version of this problem is best told as a joke. An infinite number of mathematicians walk into a bar. The first orders half a beer; the second orders a quarter; the third an eighth. After looking down the line, the bartender exclaims “You're all idiots!” pours one beer for them all to share, and closes the tab.

In this case, it’s pretty easy to see that the total of this infinite number of orders will add up to one beer. The terms in the sum get small enough quickly enough to where the total converges on some quantity.

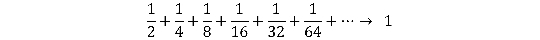
In a pie chart, it would look like this:

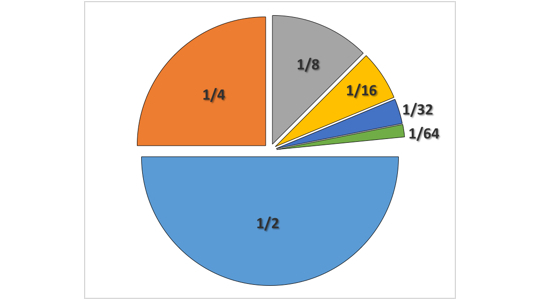
Returning to Zeno’s Paradox, let’s first get an answer using regular algebra. Setting the distance equal to x, and understanding distance to be rate × time, and that Achilles’s rate is 10 times the tortoise’s (r_t), we have the following two equations:

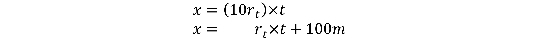
If we solve for x, we get a distance of approximately 111.11 meters. Can we calculate the distance at which Achilles will actually catch the tortoise by adding the distance between all the points where Achilles catches up to where the tortoise was before?

Yes! We can write this problem just like we did with the infinite number of mathematicians walking into a bar.

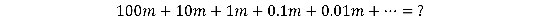
Just as before, we start by setting the unknown distance to x. We also write each term using exponents with the ratio of our runner’s speeds.

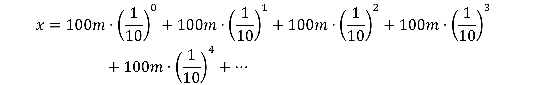
If we multiply each side by 1/10, we get the following:

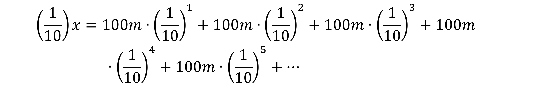
Subtracting the second equation from the first, we obtain this:

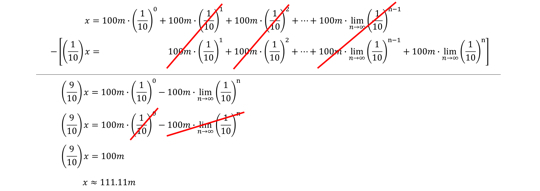
From this we see that we get exactly the same answer as before. This result is extremely important. That we can add an infinite number of things together and get a non-infinite answer is the entire basis for calculus!

Divergent series

What would happen if the tortoise instead ran twice as fast as Achilles? Achilles would again find that every time he gets to where the tortoise was before, the tortoise has moved ahead… only this time the tortoise keeps getting farther and farther away!

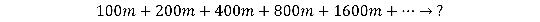
Since the numbers are getting bigger and bigger, such a series is said to be “divergent.” Setting aside how confused Achilles must be right now, let’s repeat the analysis from before just to see what happens.

As expected, it adds up to infinity. This corresponds to Achilles never catching the tortoise. To check this, what happens if we instead solve this with regular algebra?

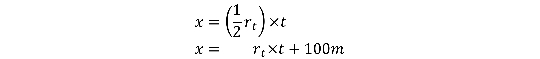
Solving for x gives a value of -100m (that’s negative 100 meters). This answer might seem strange at first, but it does actually mean something. Assuming Achilles and the tortoise were running before the start of the race, this number corresponds to the distance behind the starting line that the tortoise passed Achilles.

The really surprising thing about this is we can still use infinite series to get this answer. The folks over at MinutePhysics get a negative answer when adding an infinite number of things that sequentially get twice as big. How does this work? Is it right?

Well, yes and no. It all comes down to this part circled in green:

The green part is most certainly infinite, but strangely, we can get a meaningful answer by simply ignoring it. Make no mistake, this sum is still infinite, but by taking away the infinite part, we can still get a meaningful answer and learn things we wouldn’t be able to by doing this the “right” way.

Setting this part circled in green to zero, the final sum comes out to -100m, the same answer as before. This is what is meant by “meaningful answer.” Even though it’s not the “right” answer, this shows that there’s a way to strip away the infinite parts of a divergent series in order to get something we can glean knowledge from.