The Tendency for Order to Emerge from Chaos Was Hidden in the Most Fundamental Equations of Fluid Mechanics

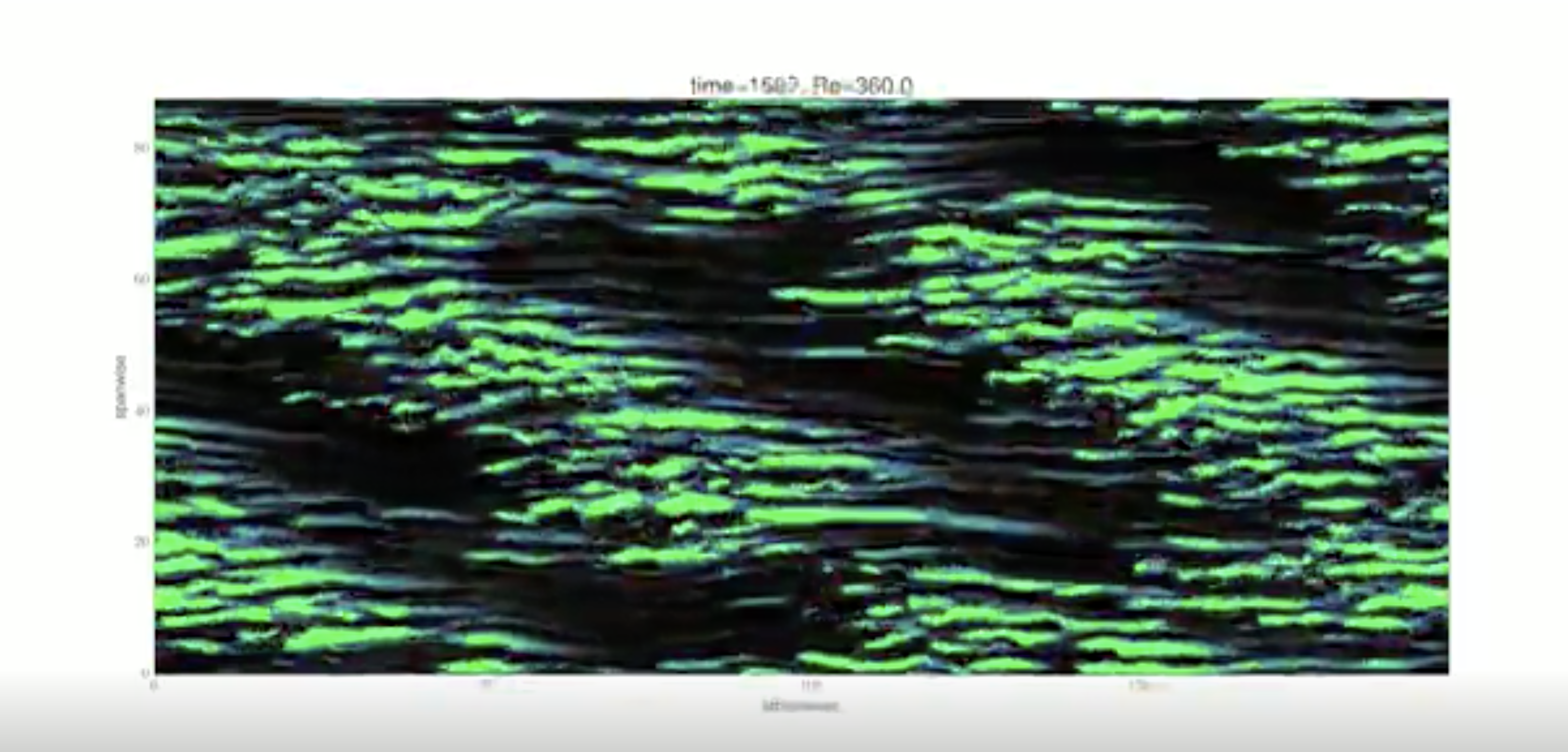
While order often devolves to chaos, sometimes the reverse is true. Turbulent fluid, for example, has a tendency to spontaneously form a tidy pattern: parallel stripes.

Though physicists had observed this phenomenon experimentally, they can now explain why this happens using fundamental fluid dynamics equations, bringing them a step closer to understanding why particles behave in this way. [The Biggest Unsolved Mysteries in Physics]

In the lab, when a fluid is placed in between two parallel plates that are moving in opposite directions from each other, its flow becomes turbulent. But after a little while, the turbulence starts to smooth out in a striped pattern. What results is a canvas of smooth and turbulent lines running at an angle to the flow (imagine slight wind-created waves in a river).

"You get structure and clear order out of the chaotic motion of turbulence," said senior author Tobias Schneider, an assistant professor in the school of engineering at the Swiss Federal Institute of Technology Lausanne. This "kind of weird and very obscure" behavior has "fascinated scientists for a long, long time."

Physicist Richard Feynman predicted that the explanation must be hidden in fundamental equations of fluid dynamics, called the Navier-Stokes equations.

But these equations are very difficult to solve and analyze, Schneider told Live Science. (Showing that the Navier-Stokes equations even have a smooth solution at every point for a 3D fluid is one of the $1 million Millennium Prize problems.) So up until this point, no one knew how the equations predicted this pattern-forming behaviors. Schneider and his team used a combination of methods, including computer simulations and theoretical calculations to find a set of "very special solutions" to these equations that mathematically describe each step of the transition from chaos to order.

In other words, they broke the chaotic behavior down into its non-chaotic building blocks and found solutions for each small chunk.  "The behavior that we observe is not mysterious physics," Schneider said. "It's somehow hidden in standard equations that describe fluid flow."

This pattern is important to understand because it shows how the turbulent and the calm, otherwise known as "laminar flow," compete with each other to determine its final state, according to a statement. When this pattern occurs, the turbulent and laminar flows are equal in strength — with no side winning the tug-of-war.

But this pattern isn't really seen in natural systems, such as turbulence in the air. Schneider notes that a pattern like this would actually "be pretty bad" for the plane because it would have to fly through a scaffold of bumpy turbulent and not turbulent lines.

Rather, the major goal of this experiment was to understand the fundamental physics of fluids in a controlled environment, he said. Only by understanding the very simple motions of fluids can we begin to understand the more complex systems of turbulence that exist everywhere around us, from the air flow around airplanes to the inside of pipelines, he added.

The researchers published their findings May 23 in the journal Nature Communications.

• The Cool Physics of 7 Classic Toys
• Image: Inside the World's Top Physics Labs
• 8 Ways You Can See Einstein's Theory of Relativity in Real Life

Originally published on Live Science.